|
|
|
Models for Decimal Tenths and Hundredths
|
|
|
|
|
|
We will consider 3 models that can be useful for helping children understand place value:
- Place value materials
- Money
- Number line
|
|
|
| Place value materials
Students are used to using PV materials to represent whole numbers. Using them to represent decimals must be approached with caution because of this. It is CRITICAL that time be spent on establishing which PV material is treated as the UNIT/ONE/WHOLE. Below three examples follow where a different PV material is the ONE.
|
|
|
|
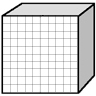
Thousands cube as the ONE
- hundreds flat becomes .1
- ten stick becomes .01
|
|
|
|
 |
|
 |
 |
 |
|
|
 |
 |
|
|
|
1.32 |
|
|
|
|
|
Hundreds flat as the ONE
- ten stick becomes .1
- unit becomes .01
|
|
 |
|
 |
 |
 |
|
|
|
1.32 |
|
|
 |
 |
|
|
|
|
|
|
Tens stick as the ONE
- unit becomes .1
- No PV material available for .01 unless you have the slice (1/10 of the unit)
|
|
|
|
 |
|
|
|
1.3 |
|
|
 |
 |
 |
|
|
|
|
|
For more detail, refer to: |
|
|
|
Grade 4 Meaning of Decimals
|
|
|
|
|
 |
|
|
|
Try these: |
 |
|
Represent 2.15 if thousands cube is the ONE |
|
|
|
 |
|
|
|
 |
|
Represent 3.04 if hundreds flat is the ONE |
|
|
|
|
|
|
Money
Do not assume that students understand decimals if they can work with money. Often students see the 'dot' in, for example, $3.15 as a separator between dollars and cents. They do not see it as having to do with place value and decimals. The following approach helps students see the connection between money and decimal tenths and hundredths.
|
|
|
| $1.32 |
 |
|
A dime is a tenth (.1). 3 dimes is .3
A penny is a hundredth (.01). 2 pennies is .02
Using, for example, a loonie, a quarter, a nickel, and two pennies is worth $1.32 but it does not connect to decimals and place value.
|
|
 |
 |
|
 |
 |
|
 |
|
|
|
|
|
$2.05 |
 |
|
 |
 |
|
 |
|
A penny is one-hundredth. 5 pennies is .05
Using a nickel to represent $.05 does not connect to decimals and place value.
|
|
|
|
 |
|
 |
|
|
|
|
 |
|
|
|
$1.10 |
 |
|
 |
|
 |
 |
|
 |
|
 |
|
A penny is one hundredth. 10 pennies is .10
Using a dime to represent .10 does not connect clearly to decimals.
.10 is ten hundredths, not one tenth
|
|
|
|
 |
 |
 |
 |
 |
|
|
|
|
$1.1 |
 |
|
|
|
A dime is one tenth or .1
Using ten pennies to represent .1 does not connect clearly to decimals.
.1 is one tenth, not ten hundredths.
|
|
|
|
 |
|
|
|
|
|
|
Try these: |
|
 |
|
Represent $1.25 using money to model decimals. |
|
|
|
 |
Represent $1.20 using money to model decimals. |
|
 |
|
|
 |
Represent $1.3 using money to model decimals. |
|
|
|
|
|
|
|
Number line
A number line is a powerful model for representing decimals. One matter to be careful about is that students do not see the modelling in a fraction way. Fractions and decimals are related but they also are very different ways to represent a part of a whole concept.
Using different language can help with this matter. Use ten-bit for tenth and ten-bit of a ten-bit for hundredth. Later on shift to decimal tenths and hundredths language.
|
|
|
|
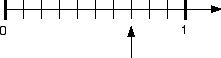
For decimal tenths, the distance between 0 and 1 (1 and 2; 2 and 3, etc,) is cut up into ten sections. This requires NINE marks (not ten marks) between the two end points. |
|
|
|
 |
|
|
|
.7 |
|
 |
|
2.7 |
|
 |
|
|
|
|
|
For decimal hundredths, the distance between 0 and .1 (.1 and .2; .2 and .3, etc,) is cut up into ten sections. This requires NINE marks (not ten marks) between the two end points. |
|
 |
|
|
|
.32 |
|
 |
|
|
|
|
|
|
|
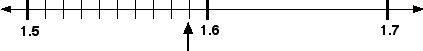
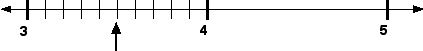
What decimal is being represented on the number line? |
|
|
|
 |
|
 |
|
|
|
|
 |
|
|
|
 |
|
 |
|
|
|
 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|